Hilbert’s Hotel takes a quantum twist
Dr Daniel Oi and Dr John Jeffers
Mathematician David Hilbert introduced a famous mathematical paradox to illustrate the confusing concept of infinity. In this scenario a hotel has an infinite number of rooms, each of which contains a guest. An infinite number of new guests all turn up at reception and ask for a room. Can the hotel accommodate them? The surprising answer is yes. If each guest already in the hotel moves to a room with room number double that of their current room number this frees up an infinite number of rooms for the new guests.
Daniel Oi and John Jeffers together with former CNQO members Vašek Potocek and Filippo Miatto in a collaboration including the Czech Technical University in Prague, Glasgow University, the University of Ottawa in Canada, the University of Rochester in New York State and the Institute for Quantum Computing in Waterloo, Canada have described the theory and performed a confirming experiment that mimics this famous paradox.
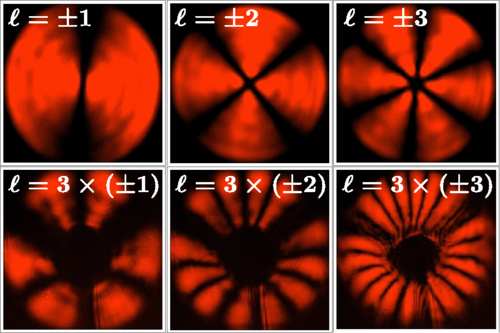
The research shows how to create gaps in a basis of quantum states by moving the state numbered by n to 3n. The extension to Hilbert’s Hotel that quantum physics allows is that not only is the occupied basis state (or room) changed, but the full complex quantum amplitude is moved to the new basis state in a coherent fashion. This means that superpositions of states with different n are maintained. The basis that is used in the experiment is the orbital angular momentum basis of light. The results reported would allow for interleaving of separate quantum states (new quantum guests). The research is reported in Physical Review Letters, PRL 115, 160505 (2015) and was highlighted by the American Physical Society http://physics.aps.org/synopsis-for/10.1103/PhysRevLett.115.160505.
19th October 2015